
Solving a logarithm without a calculation is easier than it might seem. In the exponential form, this is equivalent to 2z = 321/2 This can be rewritten as log 2 (32)1/2 = z Let us convert it to exponential form (3/2)z = (27/8) This equation is not as difficult as it may seem.

We know that 121 is 11 squared, and hence the square root of 121 is 11. To find z, first let us convert this to exponential form: 121z = 11
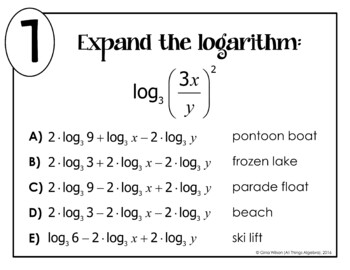
Here 64 needs to be converted to (1/4) raised to an exponent, which is the solution to the logarithm. Now let us try to find z, by simplifying the equation This can be written in another form as: 4z = 1/64 Let us consider that log 4 (1/64) equals to z Some logarithms are more complicated but can still be solved without a calculator. In such cases, it is understood that the base value by default is 10. It is to be noted that in some instances you might notice that the base is not mentioned. One the base and the number in the parenthesis are identical, the exponent of the number is the solution to the logarithm. Let us try to replace the number in the parenthesis with the base raised to an exponent. Let us use an example to understand this further: log 5 (25) Remembering and understanding this equivalency is the key to solving logarithmic problems. Here log x (y) is known as the logarithmic form, and xz = y is known as the exponential form. In other words, x needs to be raised to the power z to produce y. If xz = y, then ‘z’ is the answer to the log of y with base x, i.e., log x (y) = z The solution of any logarithm is the power or exponent to which the base must be raised to reach the number mentioned in the parenthesis. We first need to understand square, cubes, and roots of a number.
#Condense logs calculator how to#
Now, let’s get to the main part: How to Solve a Log Without Using a Calculator? The number that needs to be raised is called the base. Defining a logarithm or logĪ logarithm is defined as the power or exponent to which a number must be raised to derive a certain number. To solve a logarithm without a calculator, let us first understand what a logarithm is. The Napier constant, denoted by the letter ‘ e‘, is approximately equal to 2.718281828.Logarithms are an integral part of the calculus. The natural logarithm of a number N is the power or exponent by which ‘ e‘ must be raised to equal N. For example, a substance’s acidity and alkalinity are represented in exponential terms. These logarithms are also known as Briggsian logarithms because British mathematician Henry Briggs invented them in the 18th century. If log N = x, then this logarithmic form may be represented in exponential form, i.e., 10 x = N.Ĭommon logarithms are widely used in research and engineering. Log N or log 10 N Decadic logarithms, and decimal logarithms are other names for common logarithms. The common log of a number N is as follows: The base of a common logarithm is always 10. The natural logarithm and the common logarithm There is our Change of Base Formula, where you can learn more about the base of the logarithm and its properties. The natural logarithm has the number e as its base. The base of the logarithm is called the decimal or common logarithm.

Learn more about with our Exponent and Dividing Exponents Calculators. Here you can see that the exponential function is also used. Potentiation allows each positive number as a base to have any real power and always gives a positive result.
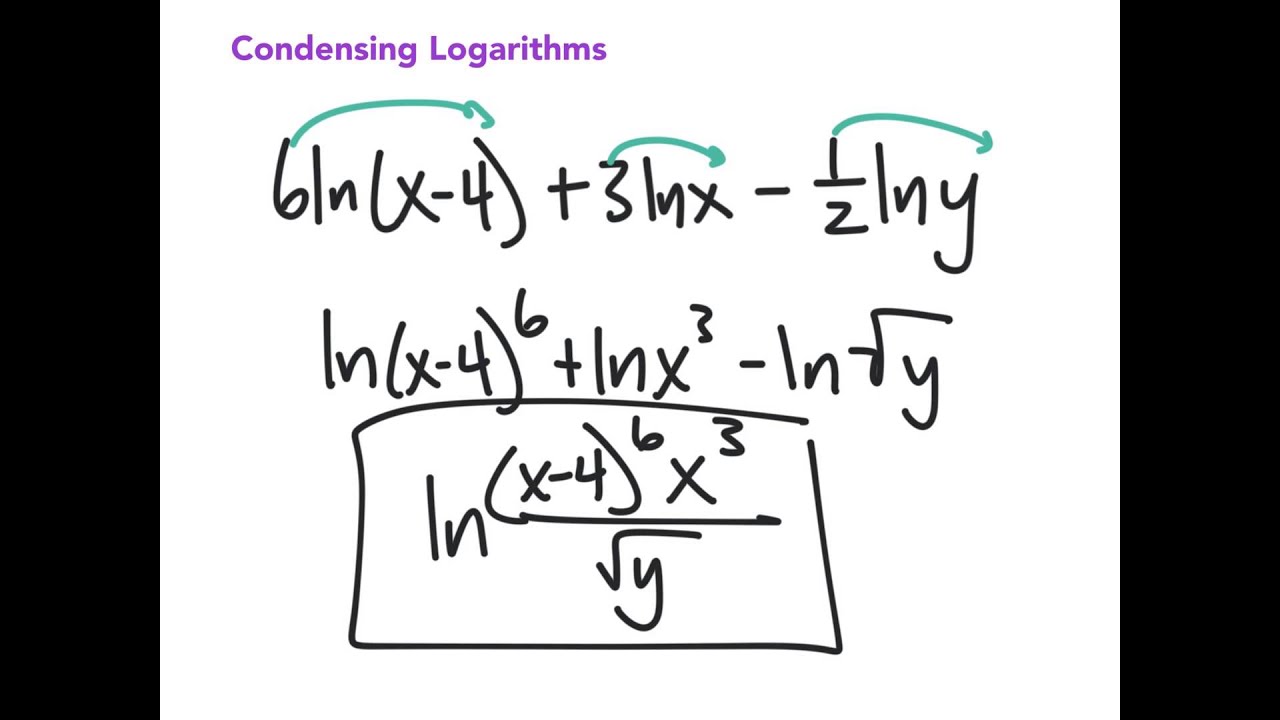
This implies that the log of a given number x is the type to which the second fixed number, base b, needs to be raised to deliver that number x. The logarithm represents the inverse function of potentiation. Fundamental counting principle calculator.Sum and difference identities calculator.Take a look other related calculators, such as: Also, to add, substract or multiply logarithms, head to Condense Logarithms Calculator, and if you want to learn more about logarithms with base 2, you can see our Log Base 2 Calculator. In the text below, we have explained the basic things about logarithms and the history of logarithms themselves. In this calculator, we will explain and make it easier for you to calculate logarithms. This Log Calculator (Logarithm) helps you to calculate the logarithm of a number with a random chosen base in the domain of a log function.


 0 kommentar(er)
0 kommentar(er)
